Order of Operations in simple Math and in Polynomials

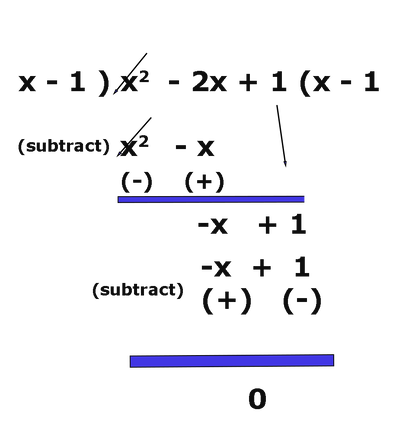
Math - Order of Operations
Order of operations is an important concept in Mathematics which sets the ground rules and a precedence. Without these ground rules, there would be different answers to one Math problem. For example, the  problem 3 + 2 * 5  can have multiple answers. The general tendency is to solve the problem from left to right. The answer using this method would be 25. Some prefer to solve moving right to left. The answer using this approach would result 13. So, What is the correct approach?
Both the solutions in the example above are wrong. Math operations must follow an order. The order is termed as ‘PEDMAS’ or ‘BEDMAS’. The order specifies that the parentheses has the top precedence, followed by Exponents, Multiplication and Division, then Addition and Subtraction.
P - Parentheses (Brackets)
E - Exponents
D - Division
M - Multiplication
A - Addition
S - Subtraction.
Following the rules of Operation in the example 3 + 2 * 6, one must multiply 2 * 6, then add the result to 3. Thus, 12 + 3 = 15. The correct answer to the problem above is 3 + 2 * 6 = 15.
The order of the operations follows a rank, with simplest of the operations taking higher precedence over other operations. Addition is the simplest. But, Multiplication is successive additions and Exponents are successive multiplications. If the operations are grouped together using parentheses or brackets, how does this affect the order?
Are the results of 2 * 2 + 3 the same as 2 * ( 2 + 3) ?  No, as the answer to the first problem is 4 + 3 = 7 is not equal to the 2 * 5 = 10.
Parentheses  has higher precedence over all the math operations. What is there are multiple parentheses?
Multiple parentheses is termed as Nested parentheses. In the example, 5 + 2 * ( 7 - 2( 6 - 4)), one must evaluate the innermost parentheses, 6 - 4 = 2. Thus,
5 + 2 *( 7 - 2 * 2)
= 5 + 2 * (7 - 4)
= 5 + 2 * 3
=  5 + 6
= 11
@YouTube - https://www.youtube.com/watch?v=dBvMiUm9aHc

The order of operations in Polynomials is combining terms  with the same exponents and solving them with math operations. Polynomial equations are solved in an order.

Polynomials
Polynomials are expressions with terms, degrees and may include numbers which are also called coefficients. Terms are some variables usually represented in the letters ‘x’, ‘y’ or ‘z’. The terms can have degrees starting from 0 and can include only whole numbers. The degrees are also called exponents. An example for a term is 6xy^2 (^ - Power) . Here, 6 is the coefficient, ‘x’ and ‘y’ are the variables. 1 and 2 are the exponents.
Polynomials are important as they are used in business, science and economics. Polynomials of degree ‘1’ is used for profit optimization in business. Polynomials of degree ‘2’ is used to solve gravity related problems in science. Polynomials of degree ‘3’ or higher is used in the field of economics.

Polynomials of degree zero are equal to one. For example, x^0  = 1. Polynomials of degree one are linear functions.For example, x + 2x = 6. Polynomials of degree two are called quadratic equations. For example, x^2 + 2x + 1.

Adding, Subtracting, Multiplying and Dividing Polynomials
For adding and subtracting the polynomials, add or subtract the coefficients of like terms. For example,
2x^2 + 3x + 2 and 3x^2 + x + 3

Add
(2 + 3) x^2  + (3 + 1) x + (2 + 3)
5x^2 + 4x + 5

Subtract
2x^2 + 3x + 2  from  3x^2 + x + 3
3x^2 + x + 3 - (2x^2 + 3x + 2)
(3 - 2)x^2 + (1 - 3)x + (3 - 2)
x^2 - 2x + 1

Multiply
When multiplying two polynomials, all the terms from one polynomial must be multiplied by all the terms from the other polynomial.
In the example x(3x - 4), x is a monomial and (3x - 4) is a binomial. By multiplying all the terms in a monomial with a binomial, the answer is 3x^2 - 4x.
In the example (x - 1)(x + 1), (x - 1) and (x + 1) are two binomials and thus multiply each term and the number of the first binomial with each term and the number of the second binomial. x*x + x*1 +(-1)*x +(1)*(-1) = x^2 + x - x - 1  =  x^2  - 1.
This process applies to multiplying polynomials with higher degrees.

Dividing Polynomials
1. Dividing a binomial with a monomial
Example,
6x^3 - 2x^2 + 4x / 2x
= 6x^3/ 2x - 2x^2 / 2x + 4x/2x
=  3x^2 - 1x + 2

2. Long Division

@YouTube -  https://www.youtube.com/watch?v=8HVtZiEpiHo

Checkout the iOS app - https://apps.apple.com/app/logarithm-exponent-polynomial/id6479218431